N displaystyle n equals the number of sides in the polygon. Therefore the sum of the interior angles of the polygon is given by the formula.
Internal And External Angles Wikipedia
Sum of interior angles of a polygon formula.
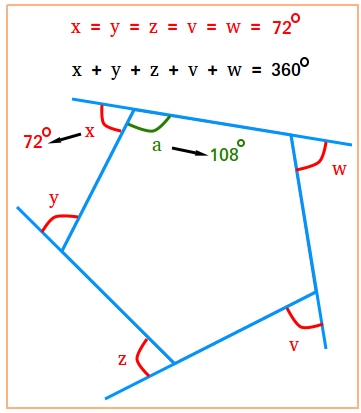
Formula for interior angles of polygons. S u m displaystyle sum is the sum of the interior angles of the polygon and. For an icosagon which is a 20-sided figure that would be. And for the square they add up to 360.
The measure of each interior angle of an equiangular n -gon is If you count one exterior angle at each vertex the sum of the measures of the exterior angles of a polygon is always 360. S u m n 2 180 displaystyle sum n-2times 180 where. Since a quadrilateral is made up of two triangles the sum of its angles would be 180 2 360.
A pentagon five-sided polygon can be divided into three triangles. Interior angles sum of polygons the angle sum of this polygon for interior angles can be determined on multiplying the. To find the sum of interior angles of a polygon multiply the number.
All the interior angles in a regular polygon are equal. The formula for finding the sum of the interior angles of a polygon is devised by the basic ideology that the sum of the interior angles of a triangle is 1800. Interior and exterior angle formulas.
Set up the formula for finding the sum of the interior angles. Lets explore interior angles of polygons. The Interior Angles of a Quadrilateral add up to 360 Because there are 2 triangles in a square.
The sum of the angles in a polygon is n-2180circ where n. Polygons are 2D shapes that have straight sides. The formula for calculating the sum of interior angles is n - 2 times 180circ where n is the number of.
Regular polygon - a polygon that has congruent angles and congruent sides. There is an interior angle at each vertex. 04 nov 2019 given an integer n the task is to find the sum of interior angles of an n sided polygon.
Sum of the Interior Angles of a Polygon 180 n-2 degrees Interior Angles of a Polygon Formula The interior angles of a polygon always lie inside the polygon. Where n is the number of sides Adjacent angles Two interior angles that share a common side are called adjacent interior angles or just adjacent angles. Posted on January 28 2021 by admin.
2 question What is the formula for the sum of the interior angle measures of a polygon. Regular polygons have sides and angles that are all the same size. In order to find the measure of a single interior angle of a regular polygon a polygon with sides of equal length and angles of equal measure with n sides we calculate the sum interior angles or n 2 180 and then divide that sum by the number of sides or n.
Interior Angles of Regular Polygons Remember that the sum of the interior angles of a polygon is given by the formula Sum of interior angles 180 n 2 where n the number of sides in the polygon. Exterior Angle Sum Property of Polygon - formula Exterior angle of polygon n 3 6 0 o. By knowing that the interior angles in a triangle add up to 180 you can calculate the.
Learn how to use a formula to find out the internal angles within a polygon. Measures of the interior angles of regular and irregular polygons. Or as a formula each interior angle of a regular polygon is given by.
The sum of interior angles in a triangle is 180. Sum Of Interior Angles Of A Polygon. Sum of Interior Angles of a Polygon Formula.
What is the measure of each individual angle in a regular icosagon a 20-sided figure. Straightedge calculator paper pencil and protractor Construct the following 5 shapes on 5 separate sheets of paper. Interior Angles of a Polygon Interior angle - an angle inside a polygon.
The sum of its angles will be 180 3 540. Now you are able to identify interior angles of polygons and you can recall and apply the formula S n 2 180 S n - 2 180 to find the sum of the interior angles of a polygon. The interior angles in a triangle add up to 180.
Interior angles of polygons The interior angles of a polygon are the angles that are inside the shape. The sum of the measures of the interior angles of a polygon with n sides is n 2180. The sum of interior angles in a quadrilateral is 360º.
Is the number of sides in the polygon.
Parallel And Perpendicular Lines Gcse Math Teaching Geometry Studying Math. Kellys Proof Statement Justification 2 4 Vertical angles are congruent.
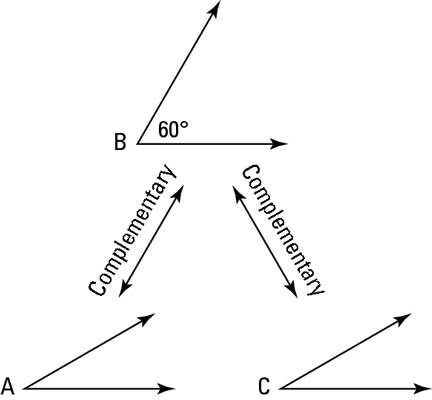 How To Prove Angles Are Complementary Or Supplementary Dummies
How To Prove Angles Are Complementary Or Supplementary Dummies
These angles arent the most exciting things in geometry but you have to be able to spot them in a diagram and know how to use the related theorems in proofs.
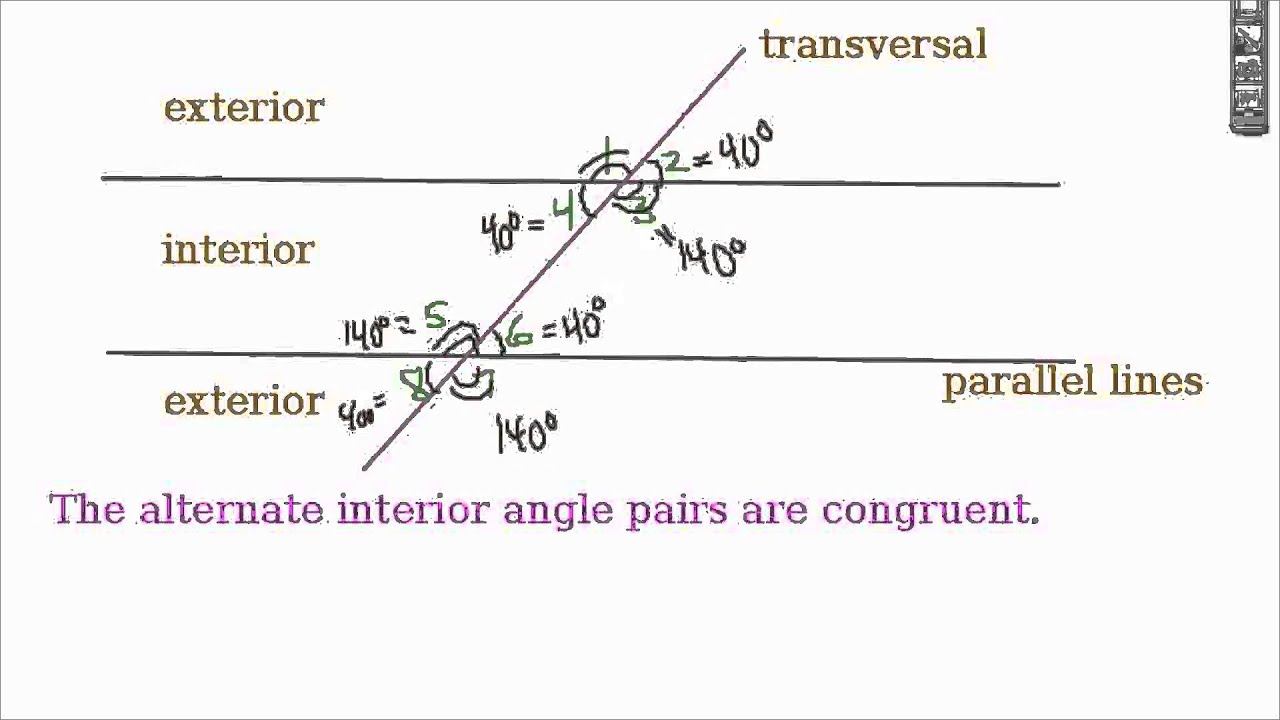
Congruent and supplementary angles. Consider the diagram below. Lets add some tools to our geometry tool belt. Reiterate how two supplementary angles make a straight angle.
Median response time is 34 minutes and may be longer for new subjects. Ashtyn and Hannah helping you with Supplementary and Congruent Angles involving Parallel lines. So let me write that down.
1 3 Vertical angles are congruent. Since angle B is supplementary to both angles C and D either of these angle measures may be used to determine the measure of Angle B. Angle DBA and angle ABC are supplementary.
Thus if one of the angle is x the other angle will be 90 x For example in a right angle triangle the two acute angles are complementary. Response times vary by subject and question complexity. The intersections of a transversal with two lines create various types of pairs of angles.
Y 1 y-3x2 -10- 2 y-3x6 1. Angles from each pair of vertical angles are known as adjacent angles and are supplementary the angles sum up to 180 degrees. Sum of the angles in a triangle is 180 degree worksheet.
For example the angles whose measures are 112 and 68 are supplementary to each other. Once again lets say that this is 50 degrees. Upon close observation its revealed that two intersecting lines give rise to four linear pairs too.
Heres a thorough explanation of complementary and supplementary angles as well as definitions of adjacent a. Sum of two complementary angles 90. In fact the only time they are congruent meaning they have the same measure is when the.
Since one angle is 90 the sum of the other two angles forms 90. Finding Supplementary Angles - Type 2. Vertical angles are congruent.
How to find supplementary angles. Play this game to review Mathematics. Ie POR ROQ 50 o 130 o 180 o.
Using the measure of either angle C or angle D we find the measure of angle B to be 180 180 x 180 180 x x. Vertical Angle Theorem Daniels Proof Statement Justification 1 2 180 Definition of Supplementary Angles 1 4 180 Definition of Supplementary Angles 1. Angle 1 27 o Find the measure of angle 4.
I already used C. Alternate Interior Angles Alternate Exterior Angles Corresponding Angles Same-Side Interior Angles supplementary. Both angle C and angle D have measures equal to 180 x and are congruent.
Powered by Create your own unique website with customizable templates. Which of the following is an equation for the line shown below. So they are supplementary.
But the angles don. Since sum of the these two angles are 180 o. Supplementary Angles Two angles are said to be supplementary to each other if sum of their measures is 180.
When two lines intersect two pairs of congruent angles are formed. Two supplementary angles add up to 180 or a straight angle. From the above example POR 50 o ROQ 130 o are supplementary angles.
Get your students to skillfully find supplementary pairs match the angles and follow the rules of the given in-out box for correct answers. If any angle of Y is less than 180 o then. It is known that AE62 AN 5b.
And are on the same side of the transversal. And lets say that this right over here is 130 degrees. So the same side interior angles.
Our printable vertical angles worksheets for grade 6 grade 7 and grade 8 take a shot at simplifying the practice of these congruent angles called vertically opposite angles. Complementary angles are two angles that add up to 90 or a right angle. Here POR is said to be supplementary angle of ROQ and ROQ is said to be supplementary angle of POR.
Clearly angle DBA plus angle ABC if you add them together you get 130 degrees plus 50 degrees which is 180 degrees. Same side interior angles are congruent. This is because in a triangle the sum of the three angles is 180.
As a consequence of Euclids parallel postulate if the two lines are parallel consecutive interior angles are supplementary corresponding angles are equal and alternate angles are equal. Same side interior angles are not always congruent. Identify the relationship of the shown pair of angles as either congruent or supplementary.
Consecutive interior angles corresponding angles and alternate angles.