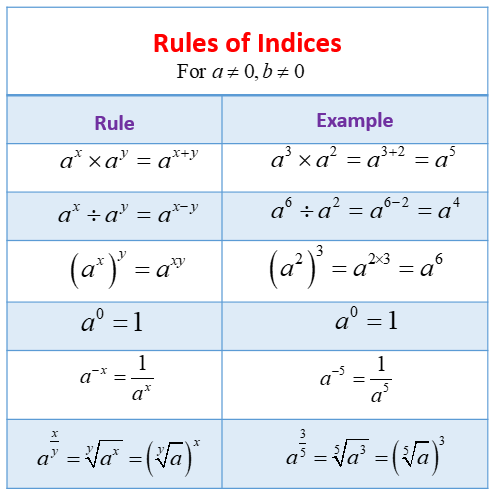
There is a focus on the reasoning behind the exponent rules as part of this practice so students will be better able to apply these rules with negative exponents. Apply the Zero-Exponent Rule.
Exponent Rules Dividing Exponents
An exponent may be referred to a number or a variable raised to another number or variable.
Division of exponents rules. The exponent rule for dividing exponential terms together is called the Quotient Rule. Power rule with radicals. Apply the Power Rule.
2 2 6 2 62 2 3 222 8. Exponentiation is a mathematical operation involving two numbers the base x and the exponent a. Whenever you divide two exponents with the same base you can simplify by subtracting the value of the exponent in the denominator by the value of the exponent in the numerator.
When a is a positive integer exponentiation corresponds to repeated multiplication of the base. Change anything raised to the zero power into a 1. For example if the base is x 2 and the exponent a 3 it is writen as x a 2 3.
M a n a n m. Here are a few examples applying the rule. Rules for multiplying and dividing.
Power of a Power Rule. B-n 1 b n. Divide two numbers with exponents by subtracting one exponent from the other.
An exponential number can be written as a n where a base and n exponent. Exponent rules are the laws or basic principles based on which problems based on exponents are solved. X 0 1.
To divide powers with the same base keep the base the same and subtract the exponents. Division is the opposite of multiplication so it makes sense that because you add exponents when multiplying numbers with the same base you subtract the exponents when dividing numbers with the same base. The exponents are commonly seen not only in mathematics but in every field.
To multiply powers with the same base keep the base the same and add the exponents. Exponents power rules Power rule I a n m a nm. Considering the division rule when we divide numbers with same base we subtract the exponents.
Dividing Fractional Exponents With the Same Base. A n m a n m Example. By using this website you agree to our Cookie Policy.
X 2 x 2 1. Free Exponents Division calculator - Apply exponent rules to divide exponents step-by-step This website uses cookies to ensure you get the best experience. 2 3 2 2 32 2 6 222222 64.
When an exponent is raised to a power multiply the exponents together. Xy z xy z. Tackle divisions of two numbers with fractional exponents by subtracting the exponent youre dividing the divisor by the one youre dividing the dividend.
Dividing zero exponents integer exponents negative exponents fractional exponents decimal exponents. Xm xn xm n. This relationship applies to dividing exponents with the same base whether the base is a number or a variable.
To divide exponents or powers with the same base subtract the exponents. Dividing exponents with different bases When the bases are different and the exponents of a and b are the same we can divide a and b first. X 1 2 x 1 2 x 1 2 1 2 x 0 1.
Zero Exponent Rule Considering several ways in which we can define an exponential number we can derive the zero-exponent rule by considering the following. 2 3 2 2 3 2 2 33 2 9 222222222 512. Division Rule Of Exponents Worksheets - there are 8 printable worksheets for this topic.
Worksheets are Exponents and division Exponent rules practic. Division or Quotient Rule. The Quotient Rule for Exponents states that when dividing exponential terms together with the same base you keep the base the same and then subtract the exponents.
Multiplication or Product Rule. Multiply or distribute the exponent outside the parenthesis with every exponent inside the parenthesis remember that if there is no exponent shown then the exponent is 1. A n b n a b n.
Review of simplifying expressions with exponents. On this lesson you will learn the exponents rule for dividing exponents with the same baseJoin us on this flipped math lesson where we visually explore div. Any number raised to the power of zero is equal to one.
If the exponential terms have multiple bases then you treat each base like a common term.
The problems in these worksheets help teach order of operations with exponents in a simple context. If it is not you must move the decimal point so that it is.
 Adding Indices Solutions Examples Videos
Adding Indices Solutions Examples Videos
You can only add and subtract exponents when they are made of like terms That means that both the base and the power must be the same.

Adding and subtracting numbers with exponents. Evaluate 3x 2 7y 2. For example take the problem 2 x2 3y x2 4 y2. Since x x 1 and 1 x 0 we can write our equation like this.
To add or subtract with powers both the variables and the exponents of the variables must be the same. How to add and subtract numbers in scientific notation with different exponents. Most interesting tasks involve unkowns but the same rules apply to them.
Xm xn xm n. In this case the two exponents 3x 2 and 7y 2 are unlike terms and so it will remain as it is. To add or subtract quantities in scientific notation numbers are manipulated so that they contain similar bases and exponents.
Once the exponents are equal then you can add or subtract the coefficients. Lets look at another example. Subtracting exponents with different base.
Canm Danm C Danm. Subtracting terms with fractional exponents follows the same rules as adding terms with fractional exponents. You add one to the exponent for each space that you move the decimal point to the left.
Here 3x and 7y both are unlike. When an exponent is raised to a power multiply the exponents together. These exponent worksheets have addition and subtraction problems adding simple exponential terms to numbers as well as adding two exponential terms to each other.
Now click the button Solve to get the sum. The same process has to be followed for subtracting numbers in scientific notation. 4 2 3 2 16 9 7.
The addition problem 22 33 becomes 2 2 3. Using this review scavenger hunt to practice the skills of multiplying and dividing monomials or in simpler terms adding and subtracting exponents. The procedure to use the adding exponents calculator is as follows.
11x 7y -2x 3x. Xm xn xm n. To subtract Polynomials first reverse the sign of each term we are subtracting in other words turn into - and - into then add as usual.
Lets look at a simple equation. Xy z xy z. One cannot add nor subtract numbers that have different exponents or different bases.
Adding and Subtracting Exponents. Finally the value of the addition of numbers with exponents will be displayed in the output field. Remember to add or subtract numbers that have exponents you must first make sure that the base and exponent of the two terms you are trying to add or subtract are the same.
X 2 3 x 1. Which of these are like terms. The terms must have the same base a and the same fractional exponent nm.
After subtracting 2xy from 2xy we ended up with 0 so there is no need to mention the xy term any more. If they are the same then all you have to do is add together their coefficients and keep the base and exponent the same. Adding and Subtracting with Exponents When dealing with numbers only we look at each expression calculate and then add or subtract as needed.
11x 2x 3x 7y. The rule is given as. Always it is easier to adjust the smaller exponent to equal the larger exponent.
Evaluate 15x 12y 11x 15x. This is done in order to ensure the corresponding integers in their coefficients are in the same place value. Multiply two numbers with exponents by adding the exponents together.
Adjust the exponents of 10 in the given numbers such that they have the same exponent. Divide two numbers with exponents by subtracting one exponent from the other. You perform the required operations on the coefficients leaving the variable and exponent as they are.
Order of Operations Factors Primes Fractions Long Arithmetic Decimals Exponents Radicals Ratios Proportions Percent Modulo Mean Median Mode Scientific Notation Arithmetics Algebra Equations Inequalities System of Equations System of Inequalities Basic Operations Algebraic Properties Partial Fractions Polynomials Rational Expressions Sequences Power Sums Pi Product Notation Induction. The final step for Adding and Subtracting in Scientific Notation is to ensure that the coefficient is between 1 and 10. This scavenger hunt has varying problems allowing all levels of students to practice and to become successful with this concept.
Enter two numbers with exponents in the respective input field. X 1 2 x 0 3 x 1 1 x 0. When adding or subtracting with powers the terms that combine always have exactly the same variables with exactly the same powers.
Do this by simplifying the fraction. The simplify calculator will then show you the steps to help you learn how to simplify your algebraic expression on your own.
 Algebra 2 Worksheets Radical Functions Worksheets Exponent Worksheets Simplifying Rational Expressions Translating Algebraic Expressions
Algebra 2 Worksheets Radical Functions Worksheets Exponent Worksheets Simplifying Rational Expressions Translating Algebraic Expressions
The negative exponents tell me to move the bases so.
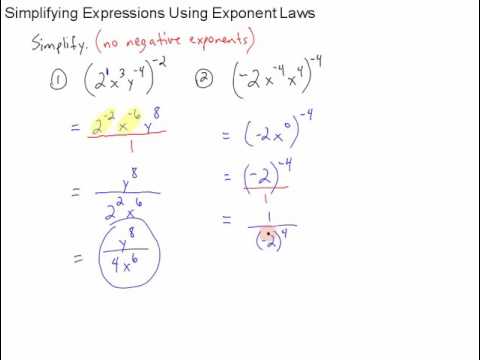
How to simplify exponents. Simplify the following expression. Mathbf color green dfrac mathit x -3 mathit x -7 x7x3. From simplify exponential expressions calculator to division we have got every aspect covered.
They mean the same thing. X 3 x 7. Combine terms with same variables and exponents.
This algebra 1 2 video tutorial shows you how to simplify radicals with variables fractions and exponents that contains both square roots cube roots an. The only difference is in the formatting. To simplify the rest of the way remember that we can make the exponent positive instead of negative by moving the resulting expression to the denominator of a fraction and setting the numerator to 1 1.
Frac 1 3 4 3. 2 2 a3 a3 b2b2 4 a6 b4 4a6b4. Power of a Power Property.
A good first step in simplifying expressions with exponents such as this is to look to group like terms together then proceed. Simplify the fraction if possible. Simplify Pre Algebra Order of Operations Factors Primes Fractions Long Arithmetic Decimals Exponents Radicals Ratios Proportions Percent Modulo Mean Median Mode Scientific Notation Arithmetics.
X 6 12 y 6 12. A Simplify 3a2b4 2ab2. 2a3b2 2 2a3b2 2a3b2.
In this video students will learn how to simplify algebraic exponents. When simplifying expressions with exponents we use the rules for multiplying and dividing exponents and negative and zero exponents. Either of the purple highlighted answers should be acceptable.
Power of a Product Property. 3 2 a2a b4b2 6 a3 b6 6a3b6. The exponent of a number says how many times to use the number in a multiplication.
Please visit our website to learn more about our organization. This algebra math video tutorial focuses on simplifying exponents with fractions variables and negative exponents including examples involving multiplicati. X 6 y 6 Solution.
B Simplify 2a3b2 2. Anything to the zero power is just 1. Rule name Rule Product Rule a m a n a m n a n b n a b n Quotient Rule a n a m a n m a n b n a b n Negative Exponent a n 1 a n Power Rule a n m a n m Tower Rule a n m a n m Fraction Rule a 1 n a n a n m a n m Zero Rule a 0 1 0 a 0 for a 0 One Rule a 1 a 1 a 1 defarraystretch15 beginarray c c text Rule name text Rule hline textProduct Rule am times an a m n a n times bn a.
3 a 12 b 6 Solution. X 6 y 6 12. This is simple enough.
For the variables I have two extra copies of xon top so the answer is. Simplifying expressions with exponents. We will begin our lesson with a review exponential form by identifying the base and order of an exponential expression and then representing each expression in expanded form.
X 6 12 y 6 12 Simplify exponents. Dfrac x -3 x -7 dfrac x7 x3 x7x3. Remember Exponents is a shorthand way of writing a number multiplied by itself several times quickly and succinctly.
Free Exponents Calculator - Simplify exponential expressions using algebraic rules step-by-step This website uses cookies to ensure you get the best experience. 8 2 8 8 64 In words. X 6 y 6 Write the square root as exponent.
Since you will be taking a root corresponding to the denominator of the exponents fraction you want the denominator to be as small as possible. If your fraction is a mixed number that is if your exponent was a decimal greater than 1 rewrite it as an improper fraction. To simplify your expression using the Simplify Calculator type in your expression like 2 5x4-3x.
8 2 could be called 8 to the second power 8 to the power 2 or simply 8 squared. X 3 y 3 x 3 y 3. X 3 x 7 x 7 x 3.
By using this website you agree to our Cookie Policy. 3 a 12 b 6. Simplifying expressions using the Laws of Exponents We can use what we know about exponents rules in order to simplify expressions with exponents.
X m x n x m n Divide two numbers with exponents by subtracting one exponent from the other. Ab n a n b n Negative exponent property.
 How To Add And Subtract With Powers Dummies
How To Add And Subtract With Powers Dummies
You can select different variables to customize these Exponents Worksheets for your needs.
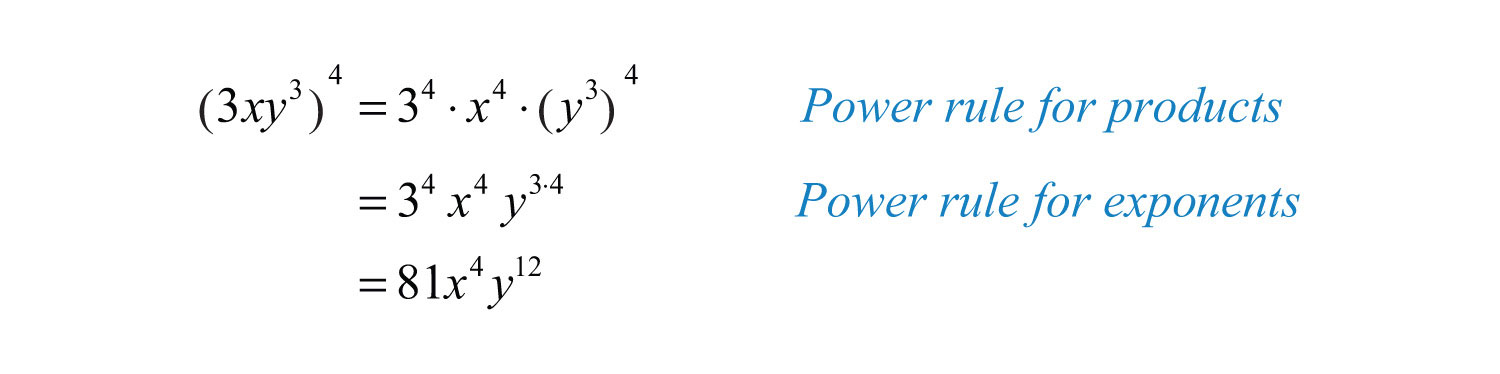
Adding exponents with variables. The basic rule in adding and subtracting variables with exponents is they must be like terms. Adding negative exponents is done by calculating each exponent first and then adding. A-m 1m and 1a-n a n.
X x x 3 x. First remember that all bases have different variables so we cant add exponents together using the Product Rule. Multiply two numbers with exponents by adding the exponents together.
With this method we can easily and quickly see the result. A n b m. Exponents are a part of algebra syllabus and a command in the basic concepts of exponents is essential for kids and students to have.
Because the variables are the same x and the powers are the same there are no exponents so the exponents must be 1 you can add the variables. Worksheet 1 Worksheet 2 Worksheet 3. Try a smart search to find answers to similar questions.
So the simplest method is to just add the exponents. Y 2 y 3 y 23 y 5. Adding exponents is done by calculating each exponent first and then adding.
Raise each part of the product to the power. The exponents tell us there are two ys multiplied by 3 ys for a total of 5 ys. In order to add or subtract variables with exponents you need to have like bases and like exponents which means that the bases and exponents are the same.
For instance when x is multiplied repeated by itself 3 times then we write this as. In that case using the Power Rule we can instead multiply the inner exponents with the outer exponent. Algebra forms one of the core areas of mathematics.
Variables are usually accompanied by coefficients. The same format of writing exponents applies with variables. Add the terms with the same base and exponent.
To make the negative exponent positive move the base and its exponent to the opposite location in the fraction. This is one of the Laws of Exponents Mixed Variables. Like terms consist of the same variable or set of variables raised to the same power.
Adding same bases b and exponents n. When we have a mix of variables just add up the exponents for each like this press play. Adding Variables With Exponents 1.
Find an answer to your question Why cant we add exponents when multiplying different variables in Mathematics if the answers seem to be not correct or theres no answer. Add the coefficients. Worksheet 1 Worksheet 2 Worksheet 3 Worksheet 4 More Addition with Exponents.
4 2 2 5 4422222 1632 48. 3 2 3 2 2 3 2 2 9 18. Adding numbers with exponents.
The numerical coefficients of these terms may vary and these are the elements that undergo the addition or subtraction process. 8 3 8 3 8 3 3 8 3 3 512 1536. Adding variables with exponents.
Variables are represented by letters and symbols. 4 2 4 2 24 2 244 32. 4 2 4 2 24 2 244 32.
The base is the large number or variable in the exponential. Because the variables are the same x and the powers are the same 2 you can perform the required operations on the variables. Algebra is broadly categorized into various fields ranging from elementary algebra that we learn during our school years to higher-order algebra like linear algebra abstract algebra and vector algebra etc.
5 2 5 2 2 5 2 2 25 50. A n m a nm. The Exponents Worksheets are randomly created and will never repeat so you have an endless supply of quality Exponents Worksheets to use in the classroom or at home.
It does not matter if the bases are. X y z x y z. Exponents Worksheets Here is a graphic preview for all of the Exponents Worksheets.
X5 y9 z23 x 5 x 3 y 9 x 3 z 2 x 3. B n b n 2b n. Adding exponents worksheets including simple problems where exponents are combined and order of operations rules PEMDAS must be observed.
Negative exponent is the reciprocal of the same expression with a positive exponent instead. X m x n x m n When an exponent is raised to a power multiply the exponents together. Power of a product.
Adding exponents with different exponents and bases. A m a nm. This algebra math video tutorial focuses on simplifying exponents with fractions variables and negative exponents including examples involving multiplicati.
When working with variables there is no way to add terms that do not. Power of a power. Find terms with the same base and the same exponent.
Multiplying exponentsAwhile multiplying two exponents with the same base the result is the same as a base expression and a new exponent created by adding the two exponents to the problem.