A regular polygon is special type of polygon. In my next note I will prove that the area of any regular polygon can be represented as.
 Regular Polygon What Is A Regular Polygon Regular Polygon Quadrilaterals Fun Math
Regular Polygon What Is A Regular Polygon Regular Polygon Quadrilaterals Fun Math
Product of the base and the height.
Area formula for a regular polygon. Equiangular is known as a regular polygon. As we know Area A ½ x p x a here p 44 cm and a 10 cm. Read our text lesson on regular p.
The side lengths of an irregular polygon are also of different measure. The perimeter is 6 x 10 n x s equal to 60 so p 60. Use the one that matches what you are given to start.
Where p the perimeter of the polygon sum of all the side lengths of a polygon. The area of the polygon is Area a x p 2 or 866 multiplied by 60 divided by 2. Equivalently it is both cyclic and equilateral or both equilateral and equiangular.
The area of a regular polygon formula is given as follows. Area of Polygon n Apothem 2 tanπn When we dont know the Apothem we can use the same formula but re-worked for Radius or for Side. Thank you for the challenge JubayerNirjhor.
The apothem is a line segment that joins the polygons center to the midpoint of any side that is perpendicular to that side. 220 cm 2. Finding the Perimeter of a Polygon Given on a Coordinate Plane.
The apothem of a regular polygon is a line segment from the centre of the polygon to the midpoint of one of its sides. And there are 2 such triangles per side or 2n for the whole polygon. An apothem is also used sometimes to find the area of a regular polygon.
½ x 44 x 10 cm 2. Find the area of a regular polygon with perimeter of 44 cm and apothem length of 10 cm. Given the length of a side.
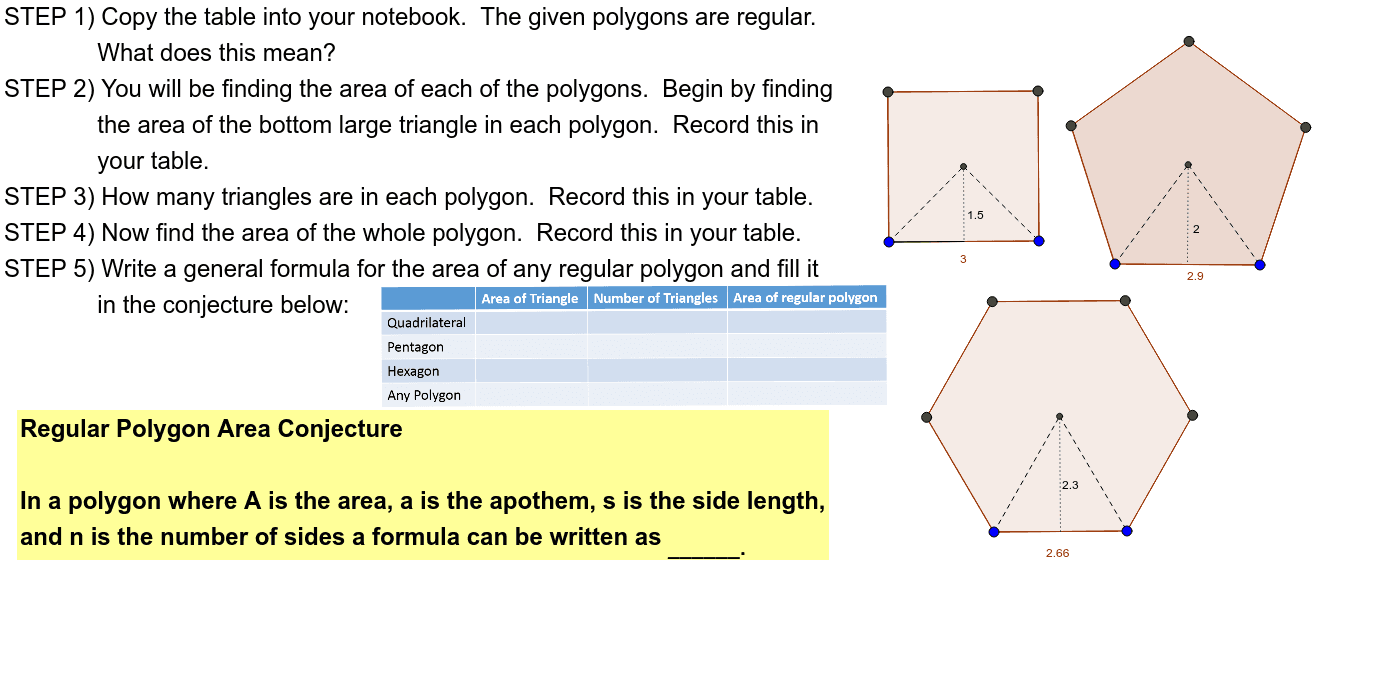
Finding the area of regular polygon when the SIDE and APOTHEM are known. Challenge in the following steps you will derive an alternate formula for finding the area of a regular polygon with n sides. A regular polygon.
Calculates side length inradius apothem circumradius area and perimeter. This MATHguide video derives the formula for the area of a regular polygon which is half the apothem times the perimeter. Your only variable will be s.
A regular polygon is equilateral it has equal sides and equiangular it has equal angles. The area of any polygon is given by. Area of a regular polygon n s a 2 n s a 2 where n n is the number of sides s s is the length of one side and a a is known as apothem it is the line from the center of the regular polygon that is perpendicular to one of its sides.
They assume you know how many sides the polygon has. Embedded content if any are copyrights of their respective owners. Area of regular polygon where p is the perimeter and a is the apothem.
If we can calculate the area of one of the triangles we can multiply by n to find the total area of the polygon. To find the area of an irregular polygon you must first separate the shape into regular polygons or plane shapes. Challenge Derive a formula for the area of a regular hexagon with sides of length s.
The result of 2tan 1806 is 11547. Therefore the area of a regular polygon is given by. The perimeter of the equilateral triangle is units.
For determining the area of a polygon given on a coordinate plane we will use the distance formula to determine the lengths of all its sides adding the lengths will give the perimeter of the polygon. Equilateral and equal angles ie. Area of Regular Polygon A regular polygon is a polygon where all the sides are the same length and all the angles are equal.
Area of a cyclic quadrilateral. In this case the hexagon has six of them. Formula for area of a regular polygon and compare it to Biancas answer.
The apothem is calculated by its own formula by plugging in 6 and 10 for n and s. The apothem is 24142 centimeters. To find the area of a regular polygon you use an apothem a segment that joins the polygons center to the midpoint of any side and that is perpendicular to that side segment HM in the following figure is an apothem.
Apothem is a segment that joins the polygons center to the midpoint of any side and it is perpendicular to that side. Here is a list of the sections within this webpage. Area of Regular Polygon.
It has 5 rectangles on the sides and 2 pentagons on the top and bottom. Therefore the area of a regular polygon is given by. A pentagonal prism 7 faces.
The solution is an area of. 4 Plug the values of a and p in the formula and get the area. Area of Polygon ½ n Radius 2 sin2 πn Area of Polygon ¼ n Side 2 tanπn A Table of Values.
Connect the dots to form the figure. The formulae below give the area of a regular polygon. Use 30-60-90 triangle ratios.
A 12. The apothem rounded to the nearest tenth is units. Area of Regular Polygon Formula A polygon having equal sides ie.
Most require a certain knowledge of trigonometry not covered in this volume but see Trigonometry Overview. Remember that the height needs to be Given ordered coordinates of a. Area ½ apothem perimeter Several other area formulas are also available.
Geometry Select an Item 2D Geometry 3D Geometry Area Chord Length Circle Circumscribed Solids Diagonal Formula Inscribed solids Perimeter Regular Polygon Surface Area Volume Perimeter of Regular Polygon can be calculated by adding the length of all sides. Plot the given coordinates. Equilateral and equal angles ie.
Therefore the area of the equilateral triangle is vor approximately 435 units The calculated areas are tan 30 n 87 rebo Then she used the formula for area of. The area of a regular polygon is given by the formula below. As shown below a regular polygon can be broken down into a set of congruent isosceles triangles.
Perimeter of Regular Polygon P. This page describes how to derive the formula for the area of a regular polygon by breaking it down into a set of n isosceles triangles where n is the number of sides.