It is why it is partial. Please watch my new version instead.
 Economics And Partial Derivatives More Examples Old Version Youtube
Economics And Partial Derivatives More Examples Old Version Youtube
A household has a utility function that they wish to maximize.
Partial derivatives in economics. Although there are examples of unconstrained optimizations in economics for example finding the optimal profit maximum revenue minimum cost etc constrained optimization is one of the fundamental tools in economics and in real life. Use of Partial Derivatives in Economics. For a multivariable function which is a continuously differentiable function the first-order partial derivatives are the marginal functions and the second-order direct partial derivatives measure the slope of the corresponding marginal functions.
Partial derivatives play a prominent role in economics in which most functions describing economic behaviour posit that the behaviour depends on more than one variable. The partial derivative with respect to y is defined similarly. It is called partial derivative of f with respect to x.
Partial derivatives If fxy is a function of two variables then x fxy is defined as the derivative of the function gx fxy where y is considered a constant. In calculating the partial derivative you are just changing the value of one variable while keeping others constant. For example a societal consumption function may describe the amount spent on consumer goods as depending on both income and wealth.
Partial derivatives usually deal with production functions and utility functions. Marginal utility of x MU_x is the first order partial derivative with respect to x. Consumers maximize their utility subject to many constraints and one significant constraint is their budget constraint.
First remember that both p and Y are treated as constants. For example if you perform the partial derivative for production function with respect to labour to find MPL this is showing how the change in labour affects the final output. Rules for finding maximisation and minimisation problems are the same as described above in case of one independent variable.
The marginal propensity to consume is then the partial derivative of the consumption function with respect to income. Another way is to say that x and y are complements if a decrease in the price of y raises the Hicksian aka compensated demand for x. Given that the utility function u fxy is a differentiable function and a function of two goods x and y.
Section 4 Use of the Partial Derivatives Marginal functions. We also use the short hand notation fxxy. HttpsyoutubeDQEQPGWILvUThis old version had poor quality sound an d the video is not great either.
Directional derivatives introduction Directional derivatives going deeper Next lesson. Therefore you treat them exactly as you would a number when. The derivative is for single variable functions and partial derivative is for multivariate functions.
Dydx measures the rate of change of y with respect to x. Partial derivatives are therefore used to find optimal solution to maximisation or minimisation problem in case of two or more independent variables. To take the partial derivative of q with respect to.
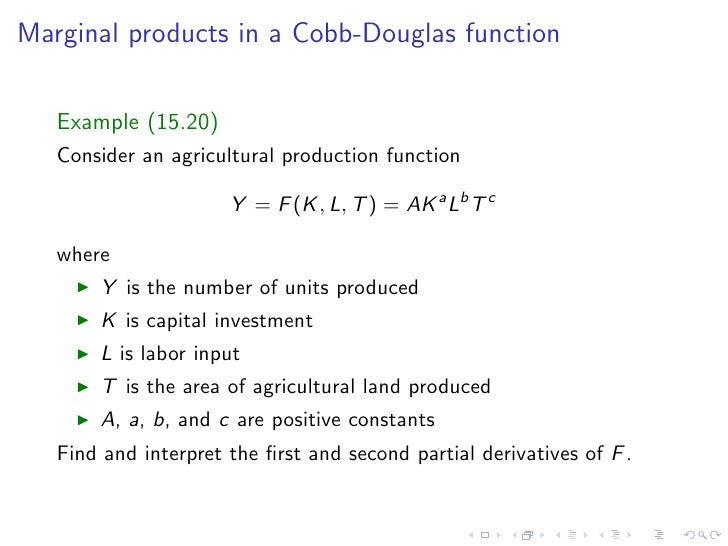
Outline Marginal Quantities Marginal products in a Cobb-Douglas function Marginal Utilities Case Study 4. Begingroup My maths knowledge is very limited but from what I understand partial derivative tells you the effect of one variable on the other. Their utility is a function of a certain bundle of goods.
In order to determine the partial derivative of quantity with respect to advertising you should take the following steps. Some Examples Marginal functions. For example if the function fxy is a continuously differentiable function.
An economic derivative is an over-the-counter OTC contract where the payout is based on the future value of an economic indicator. Reading. Use of Partial Derivatives in Economics.
This same reasoning applies for partial derivatives in economics function. The general form is z xayb and its partial derivatives are zx axa1yb and zy bxayb1 Furthermore the slope of the level curve of a Cobb-douglas is given by zx zy MRS a b y x Differentials Given the function y fx. Partial derivatives tell you how a multivariable function changes as you tweak just one of the variables in its inputAbout Khan Academy.
It is similar to other derivatives in that it is designed to. Marginal Quantities If a variable u depends on some quantity x the amount that u changes by a unit increment in x is called the marginal u of x. Partial derivative and gradient articles Introduction to partial derivatives.
Part I Partial Derivatives in Economics 3. We have learnt in calculus that when y is function of x the derivative of y with respect to x ie. This is the currently selected item.
. The Cobb-douglas function is a mathematical function that is very popular in economic models. The full derivative in this case would be the gradient.
In Economics and commerce we come across many such variables where one variable is a function of the another variable. 2 2 C p x p y 0. Since Hicksian demand is the derivative of the cost aka expenditure function by Shephards lemma this can also be expressed as a condition on mixed partials.